I will be attending the Northwest Math Conference in Whistler on Friday, October 23rd. Hope to see most of you there!
Wednesday, 30 September 2015
Tuesday, 29 September 2015
Dishes Problem- Analytical Approach
Hello Everyone!
So here is my analytical approach to this problem..
Since we have 2 guests sharing rice, 3 sharing broth and 4 sharing meat, we have think about where do they meet. What I mean by saying 'where do they meet' is that for the given scenario where there are 2+3+4= 9 total people and there are 3 dishes in total, if they rotate from one dish to other there will be 12 different combinations possible in this scenario.
To make it a little clear, please look at the following:
Multiples of 2 are: 2, 4, 6, 8, 12
Multiples of 3 are: 3, 6, 9, 12
Multiples of 4 are: 4, 8, 12
Therefore, we can see that they meet at 12.
Now, with total 12 possible combinations, 2 of them shared a bowl of rice therefore there has to be 12/2= 6 bowls of rice, 3 of them shared a bowl of broth therefore there has to be 12/3= 4 bowls of broth, and 4 of them shared a plate of meat therefore there has to be 12/4= 3 plates of meat in total.
Now you add the total number of rice bowls and broth bowls and plates of meat to find out the total number of dishes at the party:
that is- 6+4+3= 13
Now that we know that there are total number of 13 dishes with 12 people at the party.
However, we need to find number of people for 65 dishes at the party. This means 13*5 is 65, therefore, 12*5 is 60. Therefore, there is 60 total people at the party with 65 dishes present.
Cultural context has a mild effect on this problem; such as, when one is reading the problem they may get confused whether 2 guests sharing a rice bowl only eat rice or they also eat meat and broth too.
So here is my analytical approach to this problem..
Since we have 2 guests sharing rice, 3 sharing broth and 4 sharing meat, we have think about where do they meet. What I mean by saying 'where do they meet' is that for the given scenario where there are 2+3+4= 9 total people and there are 3 dishes in total, if they rotate from one dish to other there will be 12 different combinations possible in this scenario.
To make it a little clear, please look at the following:
Multiples of 2 are: 2, 4, 6, 8, 12
Multiples of 3 are: 3, 6, 9, 12
Multiples of 4 are: 4, 8, 12
Therefore, we can see that they meet at 12.
Now, with total 12 possible combinations, 2 of them shared a bowl of rice therefore there has to be 12/2= 6 bowls of rice, 3 of them shared a bowl of broth therefore there has to be 12/3= 4 bowls of broth, and 4 of them shared a plate of meat therefore there has to be 12/4= 3 plates of meat in total.
Now you add the total number of rice bowls and broth bowls and plates of meat to find out the total number of dishes at the party:
that is- 6+4+3= 13
Now that we know that there are total number of 13 dishes with 12 people at the party.
However, we need to find number of people for 65 dishes at the party. This means 13*5 is 65, therefore, 12*5 is 60. Therefore, there is 60 total people at the party with 65 dishes present.
Cultural context has a mild effect on this problem; such as, when one is reading the problem they may get confused whether 2 guests sharing a rice bowl only eat rice or they also eat meat and broth too.
Monday, 28 September 2015
Inspiring and not so inspiring Math teachers.
Hello everyone!
As most of you may already know because I have said this mostly in every class of this program throughout the week, that I am here because of a high school math teacher who helped me fall in love with math. It's because of him that I decided to become a teacher because almost every class taught by him had at least one "wow" moment that I can recall to this day. One of the best memories of mine with this teacher was when he announced in the class that whoever learns to solve a 3by3 Rubik's cube on their own and can solve it in front of him in 10 minutes will get some bonus mark on their overall mark. He knew exactly how to excite his students and motivate them to learn more and especially focus on self learning; although he was always avaiable whenever we needed him. If I can remember correctly, more than 80% of the class decided to learn how to solve the Rubik's cube and I was one of them. I taught myself using YouTube videos and getting help from my peers who knew how to solve it from before. I was able to solve it in less than 4 more minutes and he gave me the bonus marks but I felt so proud of myself for being able to do something that the majority of the people think of as a cool thing to do. I would take it everywhere I go and show it to my friends and family and feel really proud. I feel like this is part of the reason I felt like I was a mathematician and wanted to pursue that in my post secondary education. I would love to incorporate such cool ideas in my teaching career so I can inspire my students and help them find their passion for math.
Another experience that is not so inspiring was from back in the day when I was in grade 8. I studied in India till grade 10, so this experience was with a teacher from India. I used to terrible at math because I had never had a teacher who could help me see math the way I see it now. Anywho, my teacher was really fed up of me for failing almost every test she gave me that she decided to punish me for not doing well on my exams. One day I had a test and I failed it, again. As she was giving me my exam back she slapped me so hard on my face that I felt like the world was spinning around me. Not just that, she humiliated me in front of the whole class by showing my marks to everyone and told me that I cannot leave the class during the lunch break today as I have to redo the test after the break. After being humiliated and physically abused, I decided to do everything I could to pass this test. I cannot believe when I look back at this experience now that I decided to memorize the answers to the questions on the exam so I could pass the exam. I wrote the redo exam and I passed because I simply memorized the answers. It saved me from being tortured any further but at the same time I thought I had found a solution to my problem and that was to memorize the answers. This technique worked in the exam that was a make up exam for the one I failed, however, it obviously was not going to work for later exams. I failed all my exams up until I changed my school and got a teacher who had better teaching techniques. I started to gain confidence at this school and eventually got to a point where I was considered to be good at math.
As most of you may already know because I have said this mostly in every class of this program throughout the week, that I am here because of a high school math teacher who helped me fall in love with math. It's because of him that I decided to become a teacher because almost every class taught by him had at least one "wow" moment that I can recall to this day. One of the best memories of mine with this teacher was when he announced in the class that whoever learns to solve a 3by3 Rubik's cube on their own and can solve it in front of him in 10 minutes will get some bonus mark on their overall mark. He knew exactly how to excite his students and motivate them to learn more and especially focus on self learning; although he was always avaiable whenever we needed him. If I can remember correctly, more than 80% of the class decided to learn how to solve the Rubik's cube and I was one of them. I taught myself using YouTube videos and getting help from my peers who knew how to solve it from before. I was able to solve it in less than 4 more minutes and he gave me the bonus marks but I felt so proud of myself for being able to do something that the majority of the people think of as a cool thing to do. I would take it everywhere I go and show it to my friends and family and feel really proud. I feel like this is part of the reason I felt like I was a mathematician and wanted to pursue that in my post secondary education. I would love to incorporate such cool ideas in my teaching career so I can inspire my students and help them find their passion for math.
Another experience that is not so inspiring was from back in the day when I was in grade 8. I studied in India till grade 10, so this experience was with a teacher from India. I used to terrible at math because I had never had a teacher who could help me see math the way I see it now. Anywho, my teacher was really fed up of me for failing almost every test she gave me that she decided to punish me for not doing well on my exams. One day I had a test and I failed it, again. As she was giving me my exam back she slapped me so hard on my face that I felt like the world was spinning around me. Not just that, she humiliated me in front of the whole class by showing my marks to everyone and told me that I cannot leave the class during the lunch break today as I have to redo the test after the break. After being humiliated and physically abused, I decided to do everything I could to pass this test. I cannot believe when I look back at this experience now that I decided to memorize the answers to the questions on the exam so I could pass the exam. I wrote the redo exam and I passed because I simply memorized the answers. It saved me from being tortured any further but at the same time I thought I had found a solution to my problem and that was to memorize the answers. This technique worked in the exam that was a make up exam for the one I failed, however, it obviously was not going to work for later exams. I failed all my exams up until I changed my school and got a teacher who had better teaching techniques. I started to gain confidence at this school and eventually got to a point where I was considered to be good at math.
Wednesday, 23 September 2015
TIP- Exit Slip
Hello everyone!
I was not so surprised by my results for this test. I am a type of person who loves to nurture my students in every way possible. I believe in caring and connecting with students so that they can open up with you for you to guide them. I consider myself a more of a guide than a teacher because it is important to let students do inquiry and explore things under your guidance.
I was not so surprised by my results for this test. I am a type of person who loves to nurture my students in every way possible. I believe in caring and connecting with students so that they can open up with you for you to guide them. I consider myself a more of a guide than a teacher because it is important to let students do inquiry and explore things under your guidance.
However, I believe that apprenticeship is something I should have gotten a higher mark on. According to this test i don't seem to believe in apprenticeship, therefore, I would like to work on it as a teacher. I have had a chance to learn from a teacher at various occasions by learning while watching him do a certain problem. I feel obliged to incorporate apprenticeship based learning in my class as it may appeal to some students a lot.
Please see my graph attached as below:
Tuesday, 22 September 2015
Chessboard Problem
Hello Everyone!
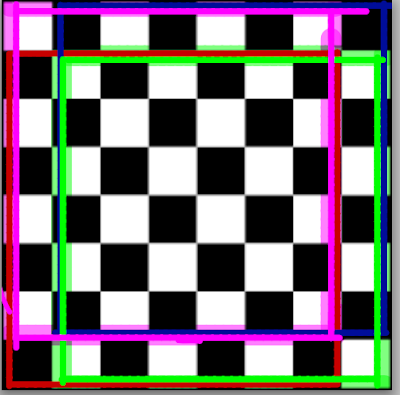
So I decided to use different colors to solve this problem. As we can all see there is a big 8 by 8 chess board that is a square itself, no one needs more explanation on that. However, it gets a little trickier when we start thinking about a 7 by 7 chess board. Now it may be a little difficult to picture a 7 by 7 chess board out of an 8 by 8 chess board; therefore, I decided to use four different colors to picture all different squares possible. As you can see below, there are 4 different ways of drawing a square that has 7 by 7 smaller squares in it (pink, red, green, blue). If we keep going further we will see that there are 9 squares for a 6 by 6 chess board out of an 8 by 8 chess board.
Therefore, as we go on we start to see a pattern and instead of counting all 200 plus squares, we use our mathematical skills to follow the pattern and fill in the blanks such as follows:
8 by 8 chess board- 1 square
7 by 7 chess board- 4 squares
6 by 6 chess board- 9 squares
5 by 5 chess board- 16 squares
4 by 4 chess board- 25 squares
3 by 3 chess board- 36 squares
2 by 2 chess board- 49 squares
1 by 1 chess board- 64 squares
Total number of squares= 1+4+9+16+25+36+49+64= 204
As a teacher, I would ask my students to find a pattern after they have solved the problem if they did not see a pattern while solving it. However, if they did see a pattern already then I would ask them to work on an 8 by 7 chess board problem.
Wednesday, 16 September 2015
Exit slip- Reflections on integrating instrumental and relational learning
Hello Everyone!
The debate on Skemp's article in today's class was full of fresh and interesting views on instrumental and relational learning. A couple of the things that were pointed out during the debate today made me think deeper about what I believed in before the debate happened.
- The 'Breaking Bad' example was one of the most powerful point views presented during the debate according to me. It made me think about the process of learning, that incorporates both instrumental and relational learning, step by step that I would like to imply in my future math classroom. This will be helpful when I start writing my lesson plans towards the end of the semester, therefore, I decided to write my response in a lesson plan form.
1) intro to the topic (both instrumental and relational learning)
2) present the formula; such as quadratic formula (instrumental learning)
3) show an example (instrumental learning)
4) get your students to practice an example with the given formula to gain confidence (instrumental learning)
5) give reasoning on why the formula works (relational learning)
6) give a small tip on the historical background of the formula (relational learning)
To summarize my thoughts on the article, before and after the debate, it is important for your students to eat the fruit of reward by learning instrumentally which will further motivate them to eat the fruit of instrumental learning.
The debate on Skemp's article in today's class was full of fresh and interesting views on instrumental and relational learning. A couple of the things that were pointed out during the debate today made me think deeper about what I believed in before the debate happened.
- The 'Breaking Bad' example was one of the most powerful point views presented during the debate according to me. It made me think about the process of learning, that incorporates both instrumental and relational learning, step by step that I would like to imply in my future math classroom. This will be helpful when I start writing my lesson plans towards the end of the semester, therefore, I decided to write my response in a lesson plan form.
1) intro to the topic (both instrumental and relational learning)
2) present the formula; such as quadratic formula (instrumental learning)
3) show an example (instrumental learning)
4) get your students to practice an example with the given formula to gain confidence (instrumental learning)
5) give reasoning on why the formula works (relational learning)
6) give a small tip on the historical background of the formula (relational learning)
To summarize my thoughts on the article, before and after the debate, it is important for your students to eat the fruit of reward by learning instrumentally which will further motivate them to eat the fruit of instrumental learning.
Tuesday, 15 September 2015
EDCP 342A- Reading Response on Richard Skemp's Instrumental vs. Relational article.
Hello Everyone!
The following three things made me stop and re-read or think a little as I was reading the article:
1) "there are two effectively different subjects being taught under the same name, 'mathematics'" (Page 6). I had to re-read this sentence in order to make sure that I read what I think I read because it is a very strong statement to make in an article as such. After reading the rest of the article I believe that it is not fair to consider two different ways of teaching as two different subjects being taught. It gives off an impression that one of the 'subjects'is right and the other has to be wrong; or both are wrong since they are complete different subjects. It would be fair to call it two different teaching styles being taught.
2) "It is easier to remember" (Page 9), under the category of the advantages of relational learning. I had to stop at this sentence as I was not sure if I fully agree with what is being said. From my personal experience as a tutor and an assistant teacher at an independent school, it really depends on the student whether they find the instrumental method to be easier or the relational method. I dealt with a student who was not willing to hear a word when it came to relational learning while he did great when he was taught instrumental learning.
3) "That relational understanding of a particular topic is too difficult, but the pupils still need it for examination reasons" (Page 11). This was one of the reasons that were given by teachers in regards to avoid relational learning in their classes. I had to stop here and think to myself that this reasoning is valid, however, there is more to this reasoning which cannot be overlooked. The students learn basic math starting kindergarten with their numbering and addition and subtraction later on. The learning in math periods that happen in most elementary schools to my knowledge is all instrumental. The students are taught that 2-2=0 but no 'whys' are asked or answered. In this case, a high school teacher in grade 8 cannot simply introduce a new method of learning math because the students are used to learning math in a particular way that is the instrumental learning.
In the end, Skemp says towards the end of the article that, "nothing else but relational understanding can ever be adequate for a teacher" (Page 11). I somewhat disagree with this statement as I believe that both instrumental and relational learning are equally important. Depending on the type of the student, the class setting and overall circumstances, either of these two types of learning should be applied. If a student has difficulty focusing on deeper issues, perhaps instrumental learning is the best way of learning for him. However, I believe that it is important to fall in love with mathematics to learn mathematics. It is fully up to the student which method helps him/her fall in love with mathematics easier and quicker. In case of instrumental learning being one's favorite way of learning will eventually make him/her eager to know all the answers to all the 'whys' he/she may have. Whereas, in case of relational learning being one's choice of learning method, they already made a wise choice by choosing the hard way of learning that comes with better rewards.
The following three things made me stop and re-read or think a little as I was reading the article:
1) "there are two effectively different subjects being taught under the same name, 'mathematics'" (Page 6). I had to re-read this sentence in order to make sure that I read what I think I read because it is a very strong statement to make in an article as such. After reading the rest of the article I believe that it is not fair to consider two different ways of teaching as two different subjects being taught. It gives off an impression that one of the 'subjects'is right and the other has to be wrong; or both are wrong since they are complete different subjects. It would be fair to call it two different teaching styles being taught.
2) "It is easier to remember" (Page 9), under the category of the advantages of relational learning. I had to stop at this sentence as I was not sure if I fully agree with what is being said. From my personal experience as a tutor and an assistant teacher at an independent school, it really depends on the student whether they find the instrumental method to be easier or the relational method. I dealt with a student who was not willing to hear a word when it came to relational learning while he did great when he was taught instrumental learning.
3) "That relational understanding of a particular topic is too difficult, but the pupils still need it for examination reasons" (Page 11). This was one of the reasons that were given by teachers in regards to avoid relational learning in their classes. I had to stop here and think to myself that this reasoning is valid, however, there is more to this reasoning which cannot be overlooked. The students learn basic math starting kindergarten with their numbering and addition and subtraction later on. The learning in math periods that happen in most elementary schools to my knowledge is all instrumental. The students are taught that 2-2=0 but no 'whys' are asked or answered. In this case, a high school teacher in grade 8 cannot simply introduce a new method of learning math because the students are used to learning math in a particular way that is the instrumental learning.
In the end, Skemp says towards the end of the article that, "nothing else but relational understanding can ever be adequate for a teacher" (Page 11). I somewhat disagree with this statement as I believe that both instrumental and relational learning are equally important. Depending on the type of the student, the class setting and overall circumstances, either of these two types of learning should be applied. If a student has difficulty focusing on deeper issues, perhaps instrumental learning is the best way of learning for him. However, I believe that it is important to fall in love with mathematics to learn mathematics. It is fully up to the student which method helps him/her fall in love with mathematics easier and quicker. In case of instrumental learning being one's favorite way of learning will eventually make him/her eager to know all the answers to all the 'whys' he/she may have. Whereas, in case of relational learning being one's choice of learning method, they already made a wise choice by choosing the hard way of learning that comes with better rewards.
Monday, 14 September 2015
Subscribe to:
Comments (Atom)