1) One of the examples that were given in this article was that in Japan, teachers ask mathematical questions differently than we ask in Canada. They ask their students, "in how many different ways can you solve this"? This question is inquiry based and can very well be used in secondary-school mathematics learning. However, the rest of the techniques that John talks about such as, asking a genuine question instead of asking as telling and not asking as asking; I will not consider them to be helpful to encourage inquiry-based learning because they are not prompting a thought process that is initiated by the students themselves. In this process, there is a big role that the teacher plays hence not really inquiry based learning. On the other hand, where John talks about intervening when the student is stuck on a problem and knowing when to intervene and how often to intervene is a great example of inquiry-based learning.
2) I had a really good short practicum where both my FA and my SA's were really happy with my first lesson. However, one of the things they told me that I need to work on is "asking questions". According to them I ask too many questions by probing them too many times. I realized right away what they were talking about and I had to fix this about my teaching. As John mentions in his article that asking as telling may not be the most efficient technique which I completely agree with. However, the biggest change that I am going to make in my unit plan after reading this article is by adding things like, "how many different solutions are possible for this problem?" or "what do you do when you get stuck" and have problems on my lesson plans where the most students will get stuck. Also, the technique of the teacher getting stuck him/herself is really impressive which I will try to use during my long practicum.
Monday, 7 December 2015
Monday, 30 November 2015
Micro teaching Reflection
My micro teaching experience was not so great. There were a few things that could have gone better as a lot of our evaluation forms talk about having a rough start with a weak hook. Also, we could have used some sort of props in our activity to make it more interesting. Doing two examples to teach the lesson was not vey effective as it came across as a lot of talking to some of our peers. We did good in terms of timing and engaging the students. I think the activity had an interesting touch to it as it was called the lifespan card activity which got people interested in it. Also, our plan did not include an extension in case we had extra time so I made that on the spot bu adding a few new things to the activity.
Lesson Plan- Micro Teaching
I had this done yesterday as a group but forgot to post it on my blog.

Teachers: Jessica, Mandeep, Simran
Topic
|
Intro to Percentages
| |
Grade level
|
8
| |
PLO
|
A3 demonstrate an understanding of percents greater than or equal to 0%
| |
Objective
|
Students will be able to use the given percentage to find the required information.
Students will be able to find percentage of two given whole numbers.
Students will have the understanding of the percentage sign.
| |
Materials
|
White board and marker. Lifespan cards.
| |
Prior Knowledge
|
Students know division and multiplication.
| |
Intro/Hook
|
4 mins
|
Percentage sign %, no calculations here.
Discuss how many pennies make up a dollar. (nickels, dimes, quarters). Each group still represents one dollar. On the board, draw out a circle representing a dollar and then fill in 25% to represent 1 quarter.
|
Development
|
4 mins
|
Finding a percent of any number with respect to another number (comparative number)
Here percent represent the fraction with denominator 100,While the number represent the amount.
Example1 - if i have 40 halloween candies ,i gave 10 % of candies to my son ,then what is the number of candies i gave to my son?
Solution-
Here, 40 is comparative number
10% of 40 candies
= 10/100* 40
= 4 candies to my son
Example 2- If i have 40 halloween candies ,i gave 10 to my daughter,then what is the percentage of the candies i gave to my daughter?
Solution-
10/40*100
=25 % of the total number of candies to my daughter
|
Activity
|
3 mins
|
-Give each student a “Lifespan” card
- Ask them to fill out the blanks on the card, their age etc
- Explain the question on the card and tell them to do their calculations on the back of the card
- let the students work in pairs of two for 2 minutes
- go around and make sure everyone's clear on the activity
|
Closure
|
1 min
|
go over the idea of percentage and how it can be used in daily life. For ex: at the shopping mall, calculating the time you have left before you have to get ready for school or dividing your food into portions and eat a certain percentage at a time. It becomes very easy to picture what's gone and what's left if you convert things into percentage.
|
----------------------------------------
BC Math 8 and 9 curriculum guide
A3 demonstrate an understanding of percents greater than or equal to 0% [CN, PS, R, V]
- provide a context where a percent may be more than 100% or between 0% and 1%
- represent a given fractional percent using grid paper represent a given percent greater than 100 using grid paper
- determine the percent represented by a given shaded region on a grid, and record it in decimal, fractional, and percent form
- express a given percent in decimal or fractional form
- express a given decimal in percent or fractional form
- express a given fraction in decimal or percent form
- solve a given problem involving percents solve a given problem involving combined percents (e.g., addition of percents, such as GST + PST)
- solve a given problem that involves finding the percent of a percent (e.g., A population increased by 10% one year and then 15% the next year. Explain why there was not a 25% increase in population over the two years.)
Sunday, 29 November 2015
Wednesday, 25 November 2015
Exit slip- video
The videos that we watched dring class were exceptionally good. A few things that stuck with me were:
- learning is now about being told, it's about exploring and getting to the point where the "aha" moment happens.
- I do not want to be a teacher; I want to be a facilitator.
- using classroom as tangibles is a great idea as kids will remember the classroom where the learning happened for the rest of their lives.
- sometimes less is more. Letting go of control can help students learn faster and better at times.
- a lesson plan should not be about what the teacher is going to do in the lesson, it should be about what the students will be doing during class and how the teacher helps them do that.
- learning is now about being told, it's about exploring and getting to the point where the "aha" moment happens.
- I do not want to be a teacher; I want to be a facilitator.
- using classroom as tangibles is a great idea as kids will remember the classroom where the learning happened for the rest of their lives.
- sometimes less is more. Letting go of control can help students learn faster and better at times.
- a lesson plan should not be about what the teacher is going to do in the lesson, it should be about what the students will be doing during class and how the teacher helps them do that.
Sunday, 22 November 2015
Arbitrary and Necessary
This
article is one of the finest articles I have read so far about teaching
Mathematics. The author says it very clearly that arbitrary is something that
students have to be informed by an external mean; whereas, necessary is
something that students can become aware of without any effort made by an
external source. Arbitrary part of your lesson is that is very definitive and
cannot be changed as it has been set by someone a long time ago. For example,
teaching students about locating hypotenuse and the opposite side on a triangle
is teaching them arbitrary part of the lesson. Necessary part of the lesson
would be something that students may discover on their own based on all they
knowledge that is given to them. For example, students may figure out on their
own that when doing fraction cancellation, one may start with any common factor
first and continue cancelling instead of always starting with the biggest
common factor.
This
article was an eye opener for me in terms of thinking, before teaching or even
planning, about what to put in your lesson and what to leave for the students
to figure out on their own. My lessons so far have been very heavy on examples.
I will be teaching one Math 8 challenge
class which I think will benefit from only learning arbitrary things from my
lesson and it would be better if I leave necessary things for them to figure
out for themselves. As the author mentions that if a teacher decides to teach
something that is necessary then a student may take it as a 'fact' and decide
to memorize it. Whereas, if this concept is taught through awareness, which is
by letting them figuring it out on their own, it would sit in their minds as a
necessary concept that they came up with on their own and they do not need to
memorize it.
Experience at the SNAP Math Fair
My experience at the SNAP Math Fair on last Wednesday was memorable one that I will always look back at and reflect upon when or if organizing a Math fair in the future. It was a fortunate and unfortunate at the same time that I could only spend time with one of the groups presenting at the fair. I failed to walk around and stop by as many projects as I could in the given time, however, I spent all my time working with one set of girls presenting a problem on patterns and its relation with Fibonacci series.
They had a set of blocks laid on the ground in a certain manner and the guest was supposed to flip it within the given number of moves. They had three different set of blocks which I was able to do fairly quickly. However, they had an extension to their problem which even they did not know the solution for. I decided to take on the challenge and started to work on the extension problem. Within minutes these two girls who were presenting the problem, got so interested in what I was trying to do that they decided to help me find the solution. I was trying to look for a pattern in the problem so that we could figure out the relation of this problem with Fibonacci series. These girls went beyond what they had in terms of supplies and used their shoes and my pen and my phone as additional number of boxes that they needed in order to find out a solution to a particular series.
Unfortunately, I was not able to find a relation between the problem and the Fibonacci series but the whole experience with the girls trying to find the solution that I will remember for the rest of my life. I was able to build a relationship with the girls bu the end of the session that they wanted to take my contact number in case I come up with a solution so I can keep them updated on that. I told them that I will get in touch with their teacher in case I come up with a solution. Overall, it was a great experience to be able to connect with these two girls and have them so interested in doing Math.
Tuesday, 17 November 2015
The SNAP Math Fair
After reading
the Math Fair booklet, it seems like a great idea for me to run a SNAP Math
Fair at my practicum high school. However, I feel like there are some
challenges that come with organizing something like this. I have three SA's for
my practicum and getting all three of them on board for this will be important
to start with. Also, the administrative will have to be in line if I were to do
this at my school. Another challenge would be to decide on a field trip
location and taking kids to the location. In spite of all these challenges I
feel very excited about doing this if all the above concerns work out in my
favor.
One thing
that I liked the most about the Snap Math Fair was that the kids have to
prepare the tabletop displays which means they are presenting the problem to
public not the solutions. This one idea seems very valuable to me. If I were to
do this at my school, I would mainly focus on getting the kids to solve the
problem, collectively, and then focus on presenting it to public and not
judges. An interesting idea would be to chose the science world to be the
location for the fair and have kids chose a science world monument that they
could relate their problem to and solve it and present it to the visitors.
Thursday, 22 October 2015
It was not quite clear in the article whether the list of "complicating factors" was a historical piece in Mathematics education or these are some of the current factors that are being faced. This area was the most fascinating and the most relatable area of the article for me.
- "mathematics is hard, cold, distant and inhuman" (Pg. 3)
I can understand that it can be hard and cold and you can feel distant about it as you may not understand its depth fully. However, how can it be inhuman? Math is inside of you and the outside of you. Your lungs move as you inhale and exhale, your brain shoots neurons as they detect an activity, you blink when your eyes get tired, all these things have a pattern. You are able to understand all these things because of math. You are able to understand the concept of balance because of math. Math cannot be inhuman. However, I will not be surprised if I get one of my students throw this term at me while they are struggling to understand something a little challenging for their brain. Something becomes inhuman when you feel beaten up after trying to understand it multiple times. This is a common feeling, it may happen while understanding chemistry or a poem or trying to make a goal at a soccer game. Any time a person feels defeated after trying over and over, they feel like the thing they are trying to achieve is inhuman. Math is comparatively a little more challenging than the other subjects taught at school which makes it the most inhuman. We, as teachers, have to be proud of the fact that we get to teach our students the most inhuman subject, not the only inhuman subject. However, it remains a question how can we make it human for them and that is a much broad subject.
- "mathematics is hard, cold, distant and inhuman" (Pg. 3)
I can understand that it can be hard and cold and you can feel distant about it as you may not understand its depth fully. However, how can it be inhuman? Math is inside of you and the outside of you. Your lungs move as you inhale and exhale, your brain shoots neurons as they detect an activity, you blink when your eyes get tired, all these things have a pattern. You are able to understand all these things because of math. You are able to understand the concept of balance because of math. Math cannot be inhuman. However, I will not be surprised if I get one of my students throw this term at me while they are struggling to understand something a little challenging for their brain. Something becomes inhuman when you feel beaten up after trying to understand it multiple times. This is a common feeling, it may happen while understanding chemistry or a poem or trying to make a goal at a soccer game. Any time a person feels defeated after trying over and over, they feel like the thing they are trying to achieve is inhuman. Math is comparatively a little more challenging than the other subjects taught at school which makes it the most inhuman. We, as teachers, have to be proud of the fact that we get to teach our students the most inhuman subject, not the only inhuman subject. However, it remains a question how can we make it human for them and that is a much broad subject.
Micro-Lesson Experience
Hello Everyone!
My Micro-lesson teaching experience was a great one. I so glad that I was able to follow the lesson plan I had come up with before class worked out so well but at the same time I was able to make a few on the spot changes which are essential for a teacher to be able to make.
I started off with the question I had planned to pose to my group but I did not know that I could get them to guess what language it was that they were looking at and then me trying to give them hints as they guessed the languages was a great addition to the hook of my lesson. I proceeded with what I had planned on earlier but I knew that I may have some extra time towards the end as we only had 4 people in our group, Ying Ting had to leave earlier. I went around the table and showed everyone how to write their name in Punjabi while telling them a little bit about alphabets and sounds of the Punjabi language. As I was doing so, Jessica asked a few questions that made me realize that I am not just a teacher at the moment, I am also a student. She asked me about the correct way of writing an alphabet in Punjabi, whether to go from bottom to top, left to right or the other way around. Her thoughtful questions added a great energy in my lesson as I had not thought about this question when I was planning my lesson. I took the challenge and taught a little bit about technicalities like this to everyone.
Towards the end of my lesson, as I had 2 minutes left and I had covered pretty much everything I had planned to, I decided to do an additional activity as I had the time for it. I asked my group whether they wanted to learn how to write their names in Hindi as well and they all looked at me with a smile and said why not. I quickly wrote their names in Hindi on their paper and told them that they can go home and practice as we won't have enough time right now. In addition to writing their names in Hindi, some asked me to write other phrases in Hindi/Punjabi for them as that might come in handy sometime in the future for them.
It was a great experience as I saw such positive attitude from all my group mates and their feedback was very helpful as well. All three of them mentioned in their feedback that this was a great lesson as they may not have gotten a chance to learn how to write their names in Hindi/Punjabi anywhere else.
Tuesday, 20 October 2015
Lesson Plan for Micro-Teaching
Hello Everyone!
I would follow the following plan for my micro-teaching lesson tomorrow in class:
30 Sec- I will have "Welcome" written on a full piece of paper in Punjabi and Hindi and I will show it to my group as we start and ask them if they know what it says?
30 Sec- How many of you know how to write your name in a language other than English? &
How many of you know how to write your name in Punjabi/Hindi? (Reminder to tell that it said "Welcome" on the sign).
20 Sec: Material: I provide them with a piece of paper and a pen each and introduce the lesson as I am distributing paper and pen
4 Min 40 Sec- Lesson: I go around the table and write their name in Punjabi on their paper (big enough and slow enough so that they can copy) while others observe how the language is written. Then I ask the person to write it 5 times or more, under where I wrote to practice while I go to the next person and write their name and so on. If I have 5 people on my table it should take me no more than 4 mins and 40 seconds to do this and I am taking account of the last person I teach how to write their name and give them time to write it 5 times. (I am 6 minutes down in my lesson at this point)
30 Sec- I will ask them if they have any questions or they need clarification on any letters and will look at their work and correct if they did not follow right. (this will be done as a group)
1 Min 30 Sec- I will ask them to turn the paper over and try to write their name in Punjabi, without looking if they can but they can look back to take hints.
1 Min- Any last minute questions and let them know that I can also write Hindi, so if they are interested in learning how to write their name in Hindi they can ask me after class.
Cool Fact: As we are wrapping up, I tell them that Punjabi is the third-most-spoken-language in Canada.
I would follow the following plan for my micro-teaching lesson tomorrow in class:
30 Sec- I will have "Welcome" written on a full piece of paper in Punjabi and Hindi and I will show it to my group as we start and ask them if they know what it says?
30 Sec- How many of you know how to write your name in a language other than English? &
How many of you know how to write your name in Punjabi/Hindi? (Reminder to tell that it said "Welcome" on the sign).
20 Sec: Material: I provide them with a piece of paper and a pen each and introduce the lesson as I am distributing paper and pen
4 Min 40 Sec- Lesson: I go around the table and write their name in Punjabi on their paper (big enough and slow enough so that they can copy) while others observe how the language is written. Then I ask the person to write it 5 times or more, under where I wrote to practice while I go to the next person and write their name and so on. If I have 5 people on my table it should take me no more than 4 mins and 40 seconds to do this and I am taking account of the last person I teach how to write their name and give them time to write it 5 times. (I am 6 minutes down in my lesson at this point)
30 Sec- I will ask them if they have any questions or they need clarification on any letters and will look at their work and correct if they did not follow right. (this will be done as a group)
1 Min 30 Sec- I will ask them to turn the paper over and try to write their name in Punjabi, without looking if they can but they can look back to take hints.
1 Min- Any last minute questions and let them know that I can also write Hindi, so if they are interested in learning how to write their name in Hindi they can ask me after class.
Cool Fact: As we are wrapping up, I tell them that Punjabi is the third-most-spoken-language in Canada.
Saturday, 17 October 2015
Big Soup Can problem
I found out online that the overall dimensions of a regular Cambell's soup can is 4 x 2.625 x 2.625 inches. If we convert this into cm, this would be 10.16 x 6.67 x 6.67 cm.
Therefore, the volume of the can is going to be pi*(radius^2)*height. Radius will be half of the diameter which is 6.67/ 2= 3.34 cm. The volume in cm would be: pi*(3.34^2)*10.16= 356.07 cm^3.
Then I searched online and found out that for Susan's high (5 feet 5 inches, as she mentioned in the class), a road bike should be around 54 cm.
By looking at the picture given of the bike in front of the big soup can, one can see that if you have 2.5 bikes stacked on top of each other in place of one bike in the picture, that would give us the approximate diameter of the big soup can. Therefore, 2.5 times the height of Susan's bike: 2.5* 54 cm= 135 cm is approximate diameter of the can.
Now we can come up with an algebraic equation as follows with the given information:
Let x= height of the big soup can.
diameter of the big soup can= 135 cm
height of the normal soup can= 10.16 cm
diameter of the normal soup can = 6.67 cm
Therefore: (135/ 6.67)* 10.16 = 205.64 cm = x
From this we can find the volume of the big soup can that is:
pi*(67.5^2)*205.64 = 2943506.60 cm^3.
Therefore,
The height of the big soup can= 205.64 cm
The diameter of the big soup can= 135 cm
Volume of the big soup can= 2943506. 60 cm
Therefore, the volume of the can is going to be pi*(radius^2)*height. Radius will be half of the diameter which is 6.67/ 2= 3.34 cm. The volume in cm would be: pi*(3.34^2)*10.16= 356.07 cm^3.
Then I searched online and found out that for Susan's high (5 feet 5 inches, as she mentioned in the class), a road bike should be around 54 cm.
By looking at the picture given of the bike in front of the big soup can, one can see that if you have 2.5 bikes stacked on top of each other in place of one bike in the picture, that would give us the approximate diameter of the big soup can. Therefore, 2.5 times the height of Susan's bike: 2.5* 54 cm= 135 cm is approximate diameter of the can.
Now we can come up with an algebraic equation as follows with the given information:
Let x= height of the big soup can.
diameter of the big soup can= 135 cm
height of the normal soup can= 10.16 cm
diameter of the normal soup can = 6.67 cm
Therefore: (135/ 6.67)* 10.16 = 205.64 cm = x
From this we can find the volume of the big soup can that is:
pi*(67.5^2)*205.64 = 2943506.60 cm^3.
Therefore,
The height of the big soup can= 205.64 cm
The diameter of the big soup can= 135 cm
Volume of the big soup can= 2943506. 60 cm
Wednesday, 14 October 2015
Borromean rings project experience
Hello Everyone!
I had the pleasure to work with a great team of three, Jordon, Pacus and myself. I was kind of nervous in the beginning as we were the only group who had the least number of people but I turned out that we three were a great team. As Susan had provided a link for the borrromean ring project, it turned out that it was the only website that had any information on the cube that we wanted to make. As soon as we realized that this was our only source with very brief description on how to make the cube, we decided to follow the only picture avaiable on the website. It was interesting how we collectively figured out the base case of the cube which was the real borromean ring of the structure. After we figured out the base case, we tried to apply the same technique to the base that we needed for the cube which involved 3 paper clips for each side, total 9 paper clips. It was difficult to work with 9 paper clips as it required a lot of force to bend them and Pearce through them. It was exciting to finally have a base for our cube that involved 9 paper clips. After this the process seemrd easy as we just had to repeat what we had done until we realized that the paper clips that we had were too small to hold a cube that had 3 layers of 3 paper clips on each side. Therefore, we ended up making a cube that was hollow from inside but had the same structure as the one that we saw online.
Along with this experience i also want to share my inquiry experience that lead me to make a shape that had 8 triangles and multiple parallelograms. I was simply playing around with paper clips using the borromean ring idea and came up with this structure. Overall, it was a very good experience and that you can come up with completely something new on our own was an incredible feeling.
I had the pleasure to work with a great team of three, Jordon, Pacus and myself. I was kind of nervous in the beginning as we were the only group who had the least number of people but I turned out that we three were a great team. As Susan had provided a link for the borrromean ring project, it turned out that it was the only website that had any information on the cube that we wanted to make. As soon as we realized that this was our only source with very brief description on how to make the cube, we decided to follow the only picture avaiable on the website. It was interesting how we collectively figured out the base case of the cube which was the real borromean ring of the structure. After we figured out the base case, we tried to apply the same technique to the base that we needed for the cube which involved 3 paper clips for each side, total 9 paper clips. It was difficult to work with 9 paper clips as it required a lot of force to bend them and Pearce through them. It was exciting to finally have a base for our cube that involved 9 paper clips. After this the process seemrd easy as we just had to repeat what we had done until we realized that the paper clips that we had were too small to hold a cube that had 3 layers of 3 paper clips on each side. Therefore, we ended up making a cube that was hollow from inside but had the same structure as the one that we saw online.
Along with this experience i also want to share my inquiry experience that lead me to make a shape that had 8 triangles and multiple parallelograms. I was simply playing around with paper clips using the borromean ring idea and came up with this structure. Overall, it was a very good experience and that you can come up with completely something new on our own was an incredible feeling.
Letters from "future ex-students"
Hello Everyone!
1) Dear Teacher,
I wanted to let you know that I have been accepted at UBC for the B.Ed. program to become a Math teacher soon. It is all your hard work that is paying off and I am where I am today. If you hadn't taught me long and difficult calculus formulas in a fun musical way that you did, I would not have been able to get through my undergrad as a Math major. Thank you for helping me fall in love with math by having faith in me that I could do better. I get to show off my math skills in front of my friends when I solve the rubik's cube in front of them. Thank you for encouraging us to learn how to solve the rubik's cube and many other cool things. Most of all, I am thankful for you to motivate me to become a Math teacher just like you and that too without ever explicitly saying it to me.
2) Dear Teacher,
I am so excited to tell you that I have been accepted at SFU in the biology program for my undergrad. I have been so excited with this news that I decided to email all of my high school teachers and give them this great news. I know this will not be super exciting for you as I am not pursuing math in my post secondary education; however, I wanted to keep you posted anyway. I know you tried your best but maybe math was not my thing. I never understood those derivatives and integrals that you taught us in the calculus class but I remember you always offering me extra help after school. So, thank you for trying even when I had decided that I cannot do math.
1) Dear Teacher,
I wanted to let you know that I have been accepted at UBC for the B.Ed. program to become a Math teacher soon. It is all your hard work that is paying off and I am where I am today. If you hadn't taught me long and difficult calculus formulas in a fun musical way that you did, I would not have been able to get through my undergrad as a Math major. Thank you for helping me fall in love with math by having faith in me that I could do better. I get to show off my math skills in front of my friends when I solve the rubik's cube in front of them. Thank you for encouraging us to learn how to solve the rubik's cube and many other cool things. Most of all, I am thankful for you to motivate me to become a Math teacher just like you and that too without ever explicitly saying it to me.
2) Dear Teacher,
I am so excited to tell you that I have been accepted at SFU in the biology program for my undergrad. I have been so excited with this news that I decided to email all of my high school teachers and give them this great news. I know this will not be super exciting for you as I am not pursuing math in my post secondary education; however, I wanted to keep you posted anyway. I know you tried your best but maybe math was not my thing. I never understood those derivatives and integrals that you taught us in the calculus class but I remember you always offering me extra help after school. So, thank you for trying even when I had decided that I cannot do math.
Wednesday, 30 September 2015
Pro- D day plans Oct 23rd
Hello Everyone!
I will be attending the Northwest Math Conference in Whistler on Friday, October 23rd. Hope to see most of you there!
Tuesday, 29 September 2015
Dishes Problem- Analytical Approach
Hello Everyone!
So here is my analytical approach to this problem..
Since we have 2 guests sharing rice, 3 sharing broth and 4 sharing meat, we have think about where do they meet. What I mean by saying 'where do they meet' is that for the given scenario where there are 2+3+4= 9 total people and there are 3 dishes in total, if they rotate from one dish to other there will be 12 different combinations possible in this scenario.
To make it a little clear, please look at the following:
Multiples of 2 are: 2, 4, 6, 8, 12
Multiples of 3 are: 3, 6, 9, 12
Multiples of 4 are: 4, 8, 12
Therefore, we can see that they meet at 12.
Now, with total 12 possible combinations, 2 of them shared a bowl of rice therefore there has to be 12/2= 6 bowls of rice, 3 of them shared a bowl of broth therefore there has to be 12/3= 4 bowls of broth, and 4 of them shared a plate of meat therefore there has to be 12/4= 3 plates of meat in total.
Now you add the total number of rice bowls and broth bowls and plates of meat to find out the total number of dishes at the party:
that is- 6+4+3= 13
Now that we know that there are total number of 13 dishes with 12 people at the party.
However, we need to find number of people for 65 dishes at the party. This means 13*5 is 65, therefore, 12*5 is 60. Therefore, there is 60 total people at the party with 65 dishes present.
Cultural context has a mild effect on this problem; such as, when one is reading the problem they may get confused whether 2 guests sharing a rice bowl only eat rice or they also eat meat and broth too.
So here is my analytical approach to this problem..
Since we have 2 guests sharing rice, 3 sharing broth and 4 sharing meat, we have think about where do they meet. What I mean by saying 'where do they meet' is that for the given scenario where there are 2+3+4= 9 total people and there are 3 dishes in total, if they rotate from one dish to other there will be 12 different combinations possible in this scenario.
To make it a little clear, please look at the following:
Multiples of 2 are: 2, 4, 6, 8, 12
Multiples of 3 are: 3, 6, 9, 12
Multiples of 4 are: 4, 8, 12
Therefore, we can see that they meet at 12.
Now, with total 12 possible combinations, 2 of them shared a bowl of rice therefore there has to be 12/2= 6 bowls of rice, 3 of them shared a bowl of broth therefore there has to be 12/3= 4 bowls of broth, and 4 of them shared a plate of meat therefore there has to be 12/4= 3 plates of meat in total.
Now you add the total number of rice bowls and broth bowls and plates of meat to find out the total number of dishes at the party:
that is- 6+4+3= 13
Now that we know that there are total number of 13 dishes with 12 people at the party.
However, we need to find number of people for 65 dishes at the party. This means 13*5 is 65, therefore, 12*5 is 60. Therefore, there is 60 total people at the party with 65 dishes present.
Cultural context has a mild effect on this problem; such as, when one is reading the problem they may get confused whether 2 guests sharing a rice bowl only eat rice or they also eat meat and broth too.
Monday, 28 September 2015
Inspiring and not so inspiring Math teachers.
Hello everyone!
As most of you may already know because I have said this mostly in every class of this program throughout the week, that I am here because of a high school math teacher who helped me fall in love with math. It's because of him that I decided to become a teacher because almost every class taught by him had at least one "wow" moment that I can recall to this day. One of the best memories of mine with this teacher was when he announced in the class that whoever learns to solve a 3by3 Rubik's cube on their own and can solve it in front of him in 10 minutes will get some bonus mark on their overall mark. He knew exactly how to excite his students and motivate them to learn more and especially focus on self learning; although he was always avaiable whenever we needed him. If I can remember correctly, more than 80% of the class decided to learn how to solve the Rubik's cube and I was one of them. I taught myself using YouTube videos and getting help from my peers who knew how to solve it from before. I was able to solve it in less than 4 more minutes and he gave me the bonus marks but I felt so proud of myself for being able to do something that the majority of the people think of as a cool thing to do. I would take it everywhere I go and show it to my friends and family and feel really proud. I feel like this is part of the reason I felt like I was a mathematician and wanted to pursue that in my post secondary education. I would love to incorporate such cool ideas in my teaching career so I can inspire my students and help them find their passion for math.
Another experience that is not so inspiring was from back in the day when I was in grade 8. I studied in India till grade 10, so this experience was with a teacher from India. I used to terrible at math because I had never had a teacher who could help me see math the way I see it now. Anywho, my teacher was really fed up of me for failing almost every test she gave me that she decided to punish me for not doing well on my exams. One day I had a test and I failed it, again. As she was giving me my exam back she slapped me so hard on my face that I felt like the world was spinning around me. Not just that, she humiliated me in front of the whole class by showing my marks to everyone and told me that I cannot leave the class during the lunch break today as I have to redo the test after the break. After being humiliated and physically abused, I decided to do everything I could to pass this test. I cannot believe when I look back at this experience now that I decided to memorize the answers to the questions on the exam so I could pass the exam. I wrote the redo exam and I passed because I simply memorized the answers. It saved me from being tortured any further but at the same time I thought I had found a solution to my problem and that was to memorize the answers. This technique worked in the exam that was a make up exam for the one I failed, however, it obviously was not going to work for later exams. I failed all my exams up until I changed my school and got a teacher who had better teaching techniques. I started to gain confidence at this school and eventually got to a point where I was considered to be good at math.
As most of you may already know because I have said this mostly in every class of this program throughout the week, that I am here because of a high school math teacher who helped me fall in love with math. It's because of him that I decided to become a teacher because almost every class taught by him had at least one "wow" moment that I can recall to this day. One of the best memories of mine with this teacher was when he announced in the class that whoever learns to solve a 3by3 Rubik's cube on their own and can solve it in front of him in 10 minutes will get some bonus mark on their overall mark. He knew exactly how to excite his students and motivate them to learn more and especially focus on self learning; although he was always avaiable whenever we needed him. If I can remember correctly, more than 80% of the class decided to learn how to solve the Rubik's cube and I was one of them. I taught myself using YouTube videos and getting help from my peers who knew how to solve it from before. I was able to solve it in less than 4 more minutes and he gave me the bonus marks but I felt so proud of myself for being able to do something that the majority of the people think of as a cool thing to do. I would take it everywhere I go and show it to my friends and family and feel really proud. I feel like this is part of the reason I felt like I was a mathematician and wanted to pursue that in my post secondary education. I would love to incorporate such cool ideas in my teaching career so I can inspire my students and help them find their passion for math.
Another experience that is not so inspiring was from back in the day when I was in grade 8. I studied in India till grade 10, so this experience was with a teacher from India. I used to terrible at math because I had never had a teacher who could help me see math the way I see it now. Anywho, my teacher was really fed up of me for failing almost every test she gave me that she decided to punish me for not doing well on my exams. One day I had a test and I failed it, again. As she was giving me my exam back she slapped me so hard on my face that I felt like the world was spinning around me. Not just that, she humiliated me in front of the whole class by showing my marks to everyone and told me that I cannot leave the class during the lunch break today as I have to redo the test after the break. After being humiliated and physically abused, I decided to do everything I could to pass this test. I cannot believe when I look back at this experience now that I decided to memorize the answers to the questions on the exam so I could pass the exam. I wrote the redo exam and I passed because I simply memorized the answers. It saved me from being tortured any further but at the same time I thought I had found a solution to my problem and that was to memorize the answers. This technique worked in the exam that was a make up exam for the one I failed, however, it obviously was not going to work for later exams. I failed all my exams up until I changed my school and got a teacher who had better teaching techniques. I started to gain confidence at this school and eventually got to a point where I was considered to be good at math.
Wednesday, 23 September 2015
TIP- Exit Slip
Hello everyone!
I was not so surprised by my results for this test. I am a type of person who loves to nurture my students in every way possible. I believe in caring and connecting with students so that they can open up with you for you to guide them. I consider myself a more of a guide than a teacher because it is important to let students do inquiry and explore things under your guidance.
I was not so surprised by my results for this test. I am a type of person who loves to nurture my students in every way possible. I believe in caring and connecting with students so that they can open up with you for you to guide them. I consider myself a more of a guide than a teacher because it is important to let students do inquiry and explore things under your guidance.
However, I believe that apprenticeship is something I should have gotten a higher mark on. According to this test i don't seem to believe in apprenticeship, therefore, I would like to work on it as a teacher. I have had a chance to learn from a teacher at various occasions by learning while watching him do a certain problem. I feel obliged to incorporate apprenticeship based learning in my class as it may appeal to some students a lot.
Please see my graph attached as below:
Tuesday, 22 September 2015
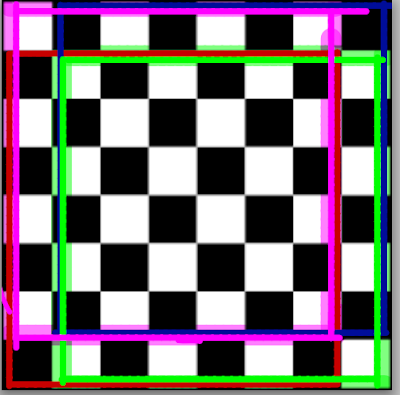
Chessboard Problem
Hello Everyone!
So I decided to use different colors to solve this problem. As we can all see there is a big 8 by 8 chess board that is a square itself, no one needs more explanation on that. However, it gets a little trickier when we start thinking about a 7 by 7 chess board. Now it may be a little difficult to picture a 7 by 7 chess board out of an 8 by 8 chess board; therefore, I decided to use four different colors to picture all different squares possible. As you can see below, there are 4 different ways of drawing a square that has 7 by 7 smaller squares in it (pink, red, green, blue). If we keep going further we will see that there are 9 squares for a 6 by 6 chess board out of an 8 by 8 chess board.
Therefore, as we go on we start to see a pattern and instead of counting all 200 plus squares, we use our mathematical skills to follow the pattern and fill in the blanks such as follows:
8 by 8 chess board- 1 square
7 by 7 chess board- 4 squares
6 by 6 chess board- 9 squares
5 by 5 chess board- 16 squares
4 by 4 chess board- 25 squares
3 by 3 chess board- 36 squares
2 by 2 chess board- 49 squares
1 by 1 chess board- 64 squares
Total number of squares= 1+4+9+16+25+36+49+64= 204
As a teacher, I would ask my students to find a pattern after they have solved the problem if they did not see a pattern while solving it. However, if they did see a pattern already then I would ask them to work on an 8 by 7 chess board problem.
Wednesday, 16 September 2015
Exit slip- Reflections on integrating instrumental and relational learning
Hello Everyone!
The debate on Skemp's article in today's class was full of fresh and interesting views on instrumental and relational learning. A couple of the things that were pointed out during the debate today made me think deeper about what I believed in before the debate happened.
- The 'Breaking Bad' example was one of the most powerful point views presented during the debate according to me. It made me think about the process of learning, that incorporates both instrumental and relational learning, step by step that I would like to imply in my future math classroom. This will be helpful when I start writing my lesson plans towards the end of the semester, therefore, I decided to write my response in a lesson plan form.
1) intro to the topic (both instrumental and relational learning)
2) present the formula; such as quadratic formula (instrumental learning)
3) show an example (instrumental learning)
4) get your students to practice an example with the given formula to gain confidence (instrumental learning)
5) give reasoning on why the formula works (relational learning)
6) give a small tip on the historical background of the formula (relational learning)
To summarize my thoughts on the article, before and after the debate, it is important for your students to eat the fruit of reward by learning instrumentally which will further motivate them to eat the fruit of instrumental learning.
The debate on Skemp's article in today's class was full of fresh and interesting views on instrumental and relational learning. A couple of the things that were pointed out during the debate today made me think deeper about what I believed in before the debate happened.
- The 'Breaking Bad' example was one of the most powerful point views presented during the debate according to me. It made me think about the process of learning, that incorporates both instrumental and relational learning, step by step that I would like to imply in my future math classroom. This will be helpful when I start writing my lesson plans towards the end of the semester, therefore, I decided to write my response in a lesson plan form.
1) intro to the topic (both instrumental and relational learning)
2) present the formula; such as quadratic formula (instrumental learning)
3) show an example (instrumental learning)
4) get your students to practice an example with the given formula to gain confidence (instrumental learning)
5) give reasoning on why the formula works (relational learning)
6) give a small tip on the historical background of the formula (relational learning)
To summarize my thoughts on the article, before and after the debate, it is important for your students to eat the fruit of reward by learning instrumentally which will further motivate them to eat the fruit of instrumental learning.
Tuesday, 15 September 2015
EDCP 342A- Reading Response on Richard Skemp's Instrumental vs. Relational article.
Hello Everyone!
The following three things made me stop and re-read or think a little as I was reading the article:
1) "there are two effectively different subjects being taught under the same name, 'mathematics'" (Page 6). I had to re-read this sentence in order to make sure that I read what I think I read because it is a very strong statement to make in an article as such. After reading the rest of the article I believe that it is not fair to consider two different ways of teaching as two different subjects being taught. It gives off an impression that one of the 'subjects'is right and the other has to be wrong; or both are wrong since they are complete different subjects. It would be fair to call it two different teaching styles being taught.
2) "It is easier to remember" (Page 9), under the category of the advantages of relational learning. I had to stop at this sentence as I was not sure if I fully agree with what is being said. From my personal experience as a tutor and an assistant teacher at an independent school, it really depends on the student whether they find the instrumental method to be easier or the relational method. I dealt with a student who was not willing to hear a word when it came to relational learning while he did great when he was taught instrumental learning.
3) "That relational understanding of a particular topic is too difficult, but the pupils still need it for examination reasons" (Page 11). This was one of the reasons that were given by teachers in regards to avoid relational learning in their classes. I had to stop here and think to myself that this reasoning is valid, however, there is more to this reasoning which cannot be overlooked. The students learn basic math starting kindergarten with their numbering and addition and subtraction later on. The learning in math periods that happen in most elementary schools to my knowledge is all instrumental. The students are taught that 2-2=0 but no 'whys' are asked or answered. In this case, a high school teacher in grade 8 cannot simply introduce a new method of learning math because the students are used to learning math in a particular way that is the instrumental learning.
In the end, Skemp says towards the end of the article that, "nothing else but relational understanding can ever be adequate for a teacher" (Page 11). I somewhat disagree with this statement as I believe that both instrumental and relational learning are equally important. Depending on the type of the student, the class setting and overall circumstances, either of these two types of learning should be applied. If a student has difficulty focusing on deeper issues, perhaps instrumental learning is the best way of learning for him. However, I believe that it is important to fall in love with mathematics to learn mathematics. It is fully up to the student which method helps him/her fall in love with mathematics easier and quicker. In case of instrumental learning being one's favorite way of learning will eventually make him/her eager to know all the answers to all the 'whys' he/she may have. Whereas, in case of relational learning being one's choice of learning method, they already made a wise choice by choosing the hard way of learning that comes with better rewards.
The following three things made me stop and re-read or think a little as I was reading the article:
1) "there are two effectively different subjects being taught under the same name, 'mathematics'" (Page 6). I had to re-read this sentence in order to make sure that I read what I think I read because it is a very strong statement to make in an article as such. After reading the rest of the article I believe that it is not fair to consider two different ways of teaching as two different subjects being taught. It gives off an impression that one of the 'subjects'is right and the other has to be wrong; or both are wrong since they are complete different subjects. It would be fair to call it two different teaching styles being taught.
2) "It is easier to remember" (Page 9), under the category of the advantages of relational learning. I had to stop at this sentence as I was not sure if I fully agree with what is being said. From my personal experience as a tutor and an assistant teacher at an independent school, it really depends on the student whether they find the instrumental method to be easier or the relational method. I dealt with a student who was not willing to hear a word when it came to relational learning while he did great when he was taught instrumental learning.
3) "That relational understanding of a particular topic is too difficult, but the pupils still need it for examination reasons" (Page 11). This was one of the reasons that were given by teachers in regards to avoid relational learning in their classes. I had to stop here and think to myself that this reasoning is valid, however, there is more to this reasoning which cannot be overlooked. The students learn basic math starting kindergarten with their numbering and addition and subtraction later on. The learning in math periods that happen in most elementary schools to my knowledge is all instrumental. The students are taught that 2-2=0 but no 'whys' are asked or answered. In this case, a high school teacher in grade 8 cannot simply introduce a new method of learning math because the students are used to learning math in a particular way that is the instrumental learning.
In the end, Skemp says towards the end of the article that, "nothing else but relational understanding can ever be adequate for a teacher" (Page 11). I somewhat disagree with this statement as I believe that both instrumental and relational learning are equally important. Depending on the type of the student, the class setting and overall circumstances, either of these two types of learning should be applied. If a student has difficulty focusing on deeper issues, perhaps instrumental learning is the best way of learning for him. However, I believe that it is important to fall in love with mathematics to learn mathematics. It is fully up to the student which method helps him/her fall in love with mathematics easier and quicker. In case of instrumental learning being one's favorite way of learning will eventually make him/her eager to know all the answers to all the 'whys' he/she may have. Whereas, in case of relational learning being one's choice of learning method, they already made a wise choice by choosing the hard way of learning that comes with better rewards.
Monday, 14 September 2015
Subscribe to:
Posts (Atom)